Back to: Geometry
Note: The sum of the angles in any triangle is equal to 180 degrees.
To find an unknown angle in any triangle if the measure of two angles are given, add them together and then subtract the sum from 180°. If two angles are the equal and their measure unknown, subtract the known angle from 180° and then divide the difference by 2.
Example 1
Find the measure of angle e.
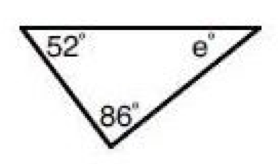
In the triangle above, one angle measures 520 and another measures 860, therefore we add 52 + 86 = 138. Subtract this from 1800, 1800 – 1380 = 420. Angle e measures 420.
Example 2
Find the measure of the missing angles.
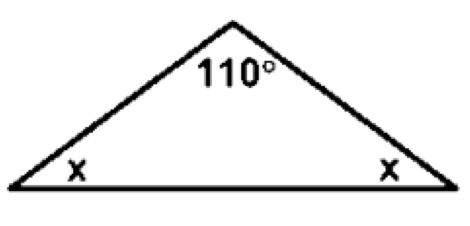
In the triangle above, one angle measures 1100 and the other two are equal and unknown. Subtract the measure of the known angle from 1800, then divide the difference by 2.
1800 – 1100 = 700. Now divide by 2, 700 ÷ 2 = 350. Each unknown angle measures 350.
Example 3
Find the value of each angle.
The sum of the angles in any triangle is equal to 180 degrees.
Therefore, in the triangle below the three unknown angles must sum to 1800.
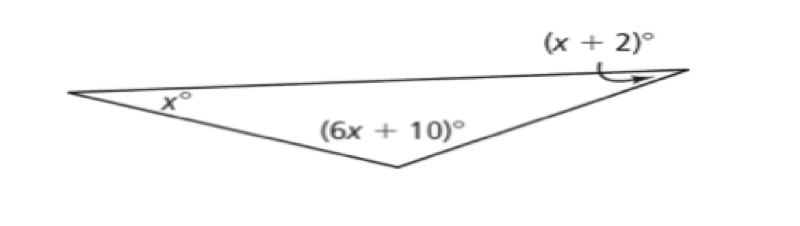
Then, x + (6x + 10) + (x + 2) = 1800
x + 6x + 10 + x + 2 = 1800
8x + 12 = 1800
– 12 – 12
——————-
8x = 1680
x = 210
6x + 10 = 6(21) + 10 = 1360
x + 2 = 21 + 2 = 230.
Solve the following problems.
Find the measure of each missing angle.
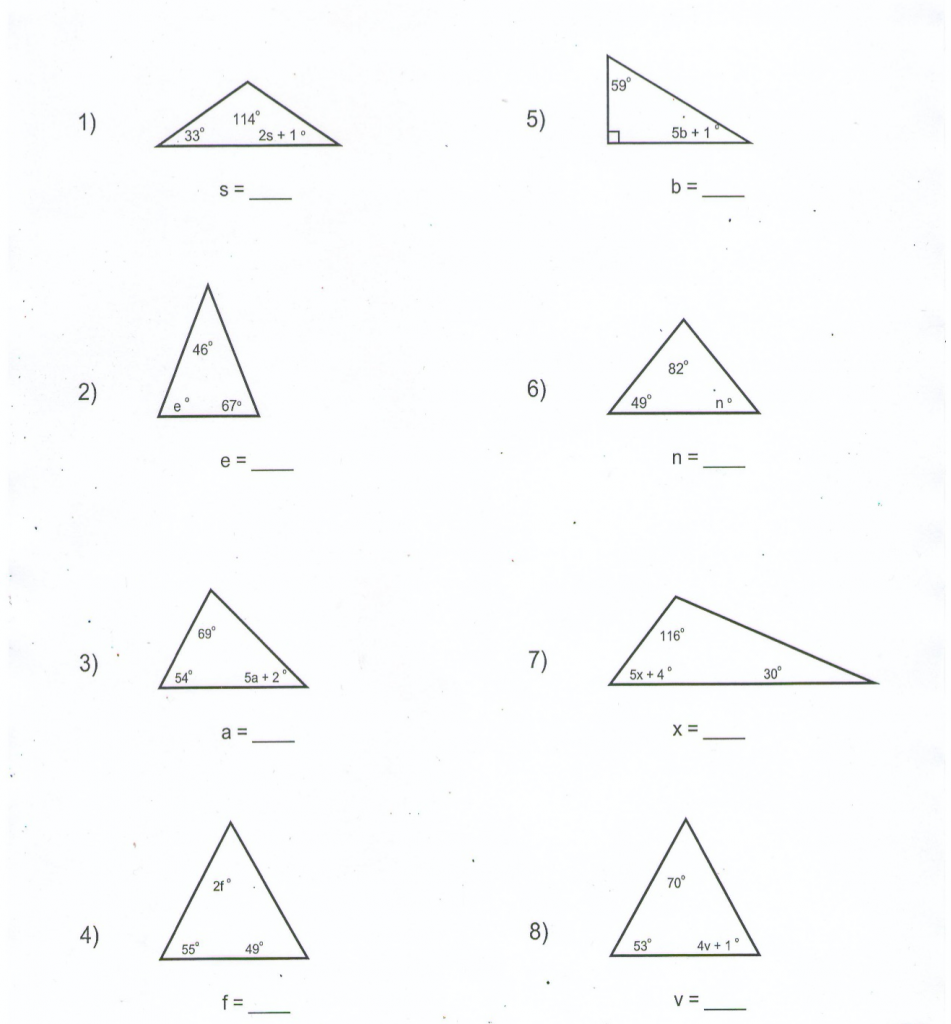
SOLUTIONS
- 16
- 67
- 11
- 38
- 6
- 49
- 6
- 14
